Halligan By The Numbers

Editor Note: The mechanical advantage of a Halligan has been a recent topic on some training forums. A friend of mine decided to sit down and put on paper what many of us explain when teaching the use of the Halligan. Before you read this I would like to start by saying this is an article for firefighters that like to keep working towards a better understanding of what they are doing and why they are doing it. Does a firefighter need to know the complete break down of mathematics regarding the leverage of the Halligan to force a door correctly? No, not at all. But a firefighter that is instructing others on how to use this tool should have a very solid grasp of how to use the different fulcrums of the tool and should be able to explain where you have more or less leverage depending on how you use it. This article allows you to take a detailed look at what the numbers can look like. This is for those of you that pay attention to the details but still understand the practical application of how this translates to actually using the tool. Understanding leverage and fulcrums is a big part of our job, during the overhaul portion of a fire ground it is very easy to see who has good understanding of tools and their leverage points, hopefully this helps bring a little further understanding to this topic. 

 Halligan By The Numbers
By Ben Shultz 

Let me start off saying that I do not hold a degree in applied mathematics, trigonometry or physics.  I do however like putting numbers and statistics to things that I do.  It’s simply what works for me and helps to solidify concepts in my mind.  So, with that said, here is a short document about the mechanical advantage that we create with the halligan.  There are some folks out there that would tell me how I forgot to factor in Pi, a cosign, theta, etc. and therefore my math isn’t perfect.  They’d be correct.  But, when we keep things simple and look at the halligan as either a class I or II lever, we can still come up with some decent numbers to show the force it can create in different applications.

Quick overview of levers…they are a simple machine.  They fall into three classes – I, II, or III.  In the case of the halligan, it operates in the class I and II mode.  Below you will see an example of a class I lever and how mechanical advantage (MA) is calculated.  Example: a seesaw.

Below here you find a class II lever and MA equation.  Example: a wheel barrow.

So, you can see in either mode, we need to know the distance from the force (the firefighter) to the fulcrum, and we need to know the distance from the load (the door) to the fulcrum.  These numbers allow us to arrive at our MA.

So, here’s what we know about the halligan before we even apply it to a door…

The halligan is 30” in overall length.

The forks are 6” and the crotch is 5”.

The adze is 6” in length.

The adze is 2” wide.

The pick is 6” in length.

The adze/ pick triangle is 5” tall.

With those numbers in mind we have most of what we need to determine how much MA we create in different positions on the door, as you will see below.

Outward Swing Doors (Towards you)

It is often advantageous to gap, crush, tunnel, etc. an outward swinging door to assist in setting the adze.  By placing the halligan in such a fashion (disregard hand position and how low on the door the halligan is placed) and moving the bar up and down we are using 30” of the bar from the fulcrum and the door is being impacted by the edge of the adze, which is 2” from the fulcrum.  Therefore, we create a 15:1 MA (a lot of force!)

Once the door is gapped, we can either drive the adze in and set it or we can drive the forks in.  I’ve heard numerous arguments for and against both methods, and that is a whole topic in itself, so for now I’ll just stick with the MA examples of each.

(Door removed for picture purposes)

In this fashion, we see the adze driven to its “mark” that lets us know we’ve driven far enough to clear the jamb and catch the door effectively.  This puts 4” of adze past the outside edge of the door frame.  That frame edge is our fulcrum.  You’ll notice that where the jamb or rabbit is located is at the 2” mark, representing where the inside door edge would be located (the load).  This equals 2” of distance from the load to the fulcrum.  Once again the distance from the force to the fulcrum is 30”, giving us a 15:1 MA.

Here we have placed the forks for the outward swinging door.  Once again, there could be a entire discussion devoted to bevel away or towards a door in forcible entry, but we’re just sticking to MA for now.  Note that the fulcrum (outside edge of door frame) is created at about the 3.5” mark.  The jamb is located at the 1.5” mark.  Once again our load to fulcrum distance is 2”.  However, in the forks configuration, we lose a bit of MA, because our force to fulcrum distance is reduced by the length of fork  we drive past the fulcrum, in this case that is 3.5”.  Therefore we end up with 30” bar minus 3.5” for a force to fulcrum length of 26.5”.  So our MA is 26.5 / 2, which equals 13.25:1, still significant, but reduced from the adze application.  What’s important to note is that if we had driven the forks to the crotch mark, as is often taught, we reduce our MA to 25/2, or 12.5:1.  I know the concept of driving the forks to the crotch is to assure that you have cleared the jamb and have enough fork to grab the door, but it is obvious from this picture that those concerns are addressed with a shallower drive.  It’s something to think about.

Inward Swinging Doors (Away from you)

Here we see the set up to initially gap (and possibly force) the inward swinging door.  The halligan is used in the same fashion as gapping the outward swinging door, just in a different position.  By pushing down on the bar and forks we create a fulcrum on the edge of the 2” wide adze, therefore, the math doesn’t change and we have a 15:1 MA.  It’s important to realize that pulling up in this configuration (right side opening door)  greatly decreases your MA due to the length of the pick.

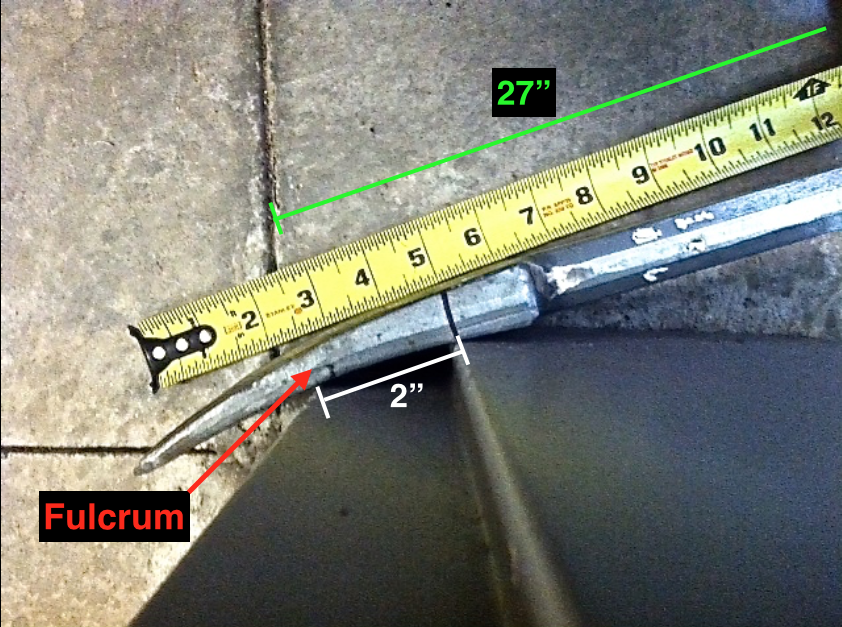
Up until now, the halligan has been used in the class I lever mode.  Here we find it used in the class II mode.  In this set up, we have driven the forks to the crotch mark.  This creates our fulcrum at the edge of the door frame at the 3” mark and puts our load at the jamb at the 5” mark, once again, giving us a load to fulcrum length of 2”.  Because we have driven our forks 3” past our door frame edge (fulcrum), we have also reduced our force to fulcrum length by 3”, resulting in a D(f) of 27”.  Therefore our MA is 27 / 2, or 13.5:1.  Once again, I know it is often taught to drive to the crotch, but remember, the deeper we drive the forks, the less MA we have available to us.

I’m sure with the 90 degree bend in a halligan bar and the curve of the forks that there are some really fancy equations to truly figure out the exact MAs for the halligan.  So, if you’re not entirely thrilled with the numbers as fact, consider them arbitrary values and simply think of the different configurations as “more” or “less” MA.  The key is to have a good grasp on the capabilities of the tool.  And don’t forget, in order to achieve the greatest mechanical advantage you have to maximize the force to fulcrum distance, meaning you must pull or push at the end of the halligan.  The closer you move to the door, the less MA you realize.  With that said, remember that you can increase your force to fulcrum distance, therefore increasing your MA, by introducing a roof hook into the mix or marrying up another halligan at the forks.  Finally, like most things in life, there is a trade-off involved.  The greater your MA, the shorter your “throw” when forcing a door and vice versa.

(Editor Conclusion)

I first saw this “ballpark mechanical advantage explanation” from Josh Materi while he was explaining Halligan use in a hands on forcible entry drill (video below). Josh does a good job explaining a couple of other ways we commonly use the tool that is not included in the numbers above. What you have to keep in mind is as you force a door and material starts moving, fulcrums change and so does the point of the tool that is prying on the door. This is what makes this rough math and a constantly changing mechanical advantage but you get the picture.  Josh breaks down the adze end of the tool into three major categories (the 2″ adze, the 6″ adze and the pike/adze combo) This is the easiest way I have found to keep it simple when instructing which part means what when we put it to work on a door. Thanks a ton to Ben Shultz for writing this article and Josh Materi for permission to use his video clip. -Royal